Consider problems 10-11 (13.3) in Larson’s 9e calculus textbook:
A student just beginning to work with partial derivatives might wonder why, in the partial derivative with respect to x in problem 10, y vanishes, whereas, in problem 11, y3 remains even when we differentiate with respect to x. Here’s the key difference. In the function in problem 11, we are multiplying x2 by y3. In the function in problem 10, we were subtracting the y term from the x term. In problem 11, think of y3 as a constant that is multiplying x. Imagine that, in place of y3, you have simply 7. If you had 7x2, your derivative would be 14x. In other words, we would multiply by 7; the 7 wouldn’t go anywhere. In problem 11, y3 is a constant that multiplies x, so we must retain it, which is why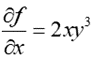 
. If the y term were being added to or subtracted from the x term, then in the partial derivative with respect to x, the y term would indeed vanish, as the constants would become 0 when differentiated with respect to x. On the other hand, because y3 as a constant is multiplying the x term in problem 11, it will remain as is when differentiating with respect to x.
BridgeText can help you with all of your statistical analysis and mathematical needs.
