When dealing with position, velocity, acceleration, and distance in calculus, you're typically working with functions that describe the motion of an object. These functions are related through differentiation and integration. Here's how you can relate them:
Position
This is usually described by a function s(t) or x(t), where t is time.
Velocity
The velocity function, v(t), is the derivative of the position function. To find it, you take the first derivative of the position function with respect to time.
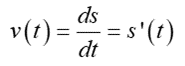
Acceleration
The velocity function, v(t), is the derivative of the velocity function. It’s also the second derivative of the position function:
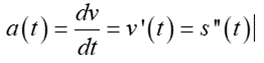
Distance
For distance, you integrate the absolute value of the velocity function:
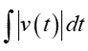
Linking Concepts
Position -> Velocity -> Acceleration: Think of it as increasing levels of "change." Position tells you where you are, velocity tells you how your position is changing, and acceleration tells you how your velocity is changing. You differentiate as you move down this chain.
Acceleration -> Velocity -> Position: Conversely, if you want to go from acceleration to velocity or velocity to position, you integrate. You can remember this by thinking that acceleration is the "cause" and position is the "effect." You're going from cause to effect, and to get there, you integrate.
Here's a handy mnemonic using the first letter of each term:
- Position
- Velocity
- Acceleration
- Distance
You could remember "People View A Dance" or some other mnemonic using those initial letters.
To summarize:
- To go from position to velocity, or from velocity to acceleration, you differentiate.
- To go from acceleration to velocity, or from velocity to position, you integrate.
- Finding the total distance traveled involves integrating the absolute value of the velocity.





