Very few people are familiar with the concept of kurtosis, but, in quantitatively oriented academic papers, theses, or research essays, kurtosis is a property that you have to understand, generate (through the use of statistical programs), and interpret.
Kurtosis is a measurement of the extent to which the distribution of a ratio or interval variable is peaked. Let’s look at kurtoses above and below 3, given that 3 is the kurtosis of a standard normal distribution.
Kurtosis > 3
Imagine that, in a sample of 1,000,000 people, the majority make $40,000 a year, with very little variation around that sum.
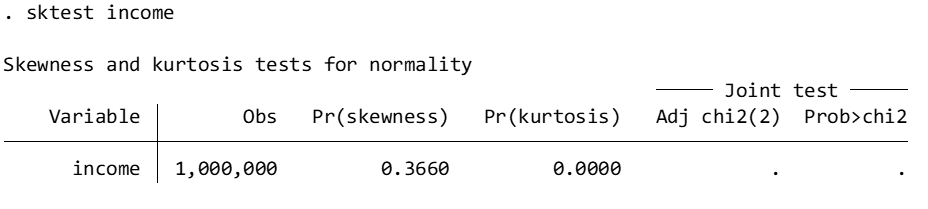
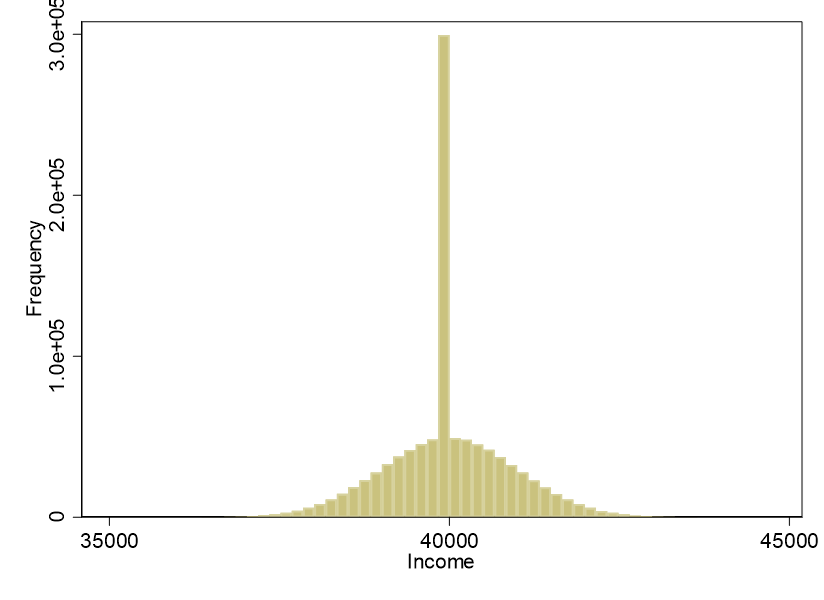This distribution has a kurtosis of 4, which is quite high. A high kurtosis will have a high peak (or more than one high peak), of which the histogram above provides a simple example. Notably, the standard normal distribution has a kurtosis of 3. If your kurtosis is notably higher (or lower) than 3, one option you have is to test the normality of your kurtosis. I used Stata for this purpose and found that the kurtosis of 4 in the histogram above is not normal, p < .0001 (note that the skewness is normal, as p > .05).
Kurtosis < 3
Here is a completely different distributional pattern of the same variable, one in which there is a flat-top distribution without a peak. In this distribution, every participant was roughly equally likely to have an income between $30,000 and $50,000. On the other hand, in our previous histogram, a lot of people made exactly $40,000.
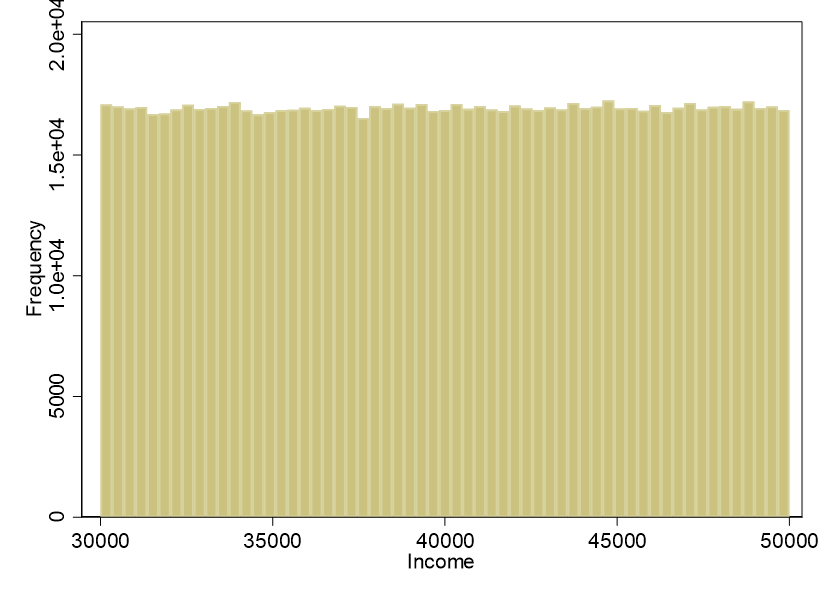The kurtosis of the distribution portrayed above is 1.80, and it deviates from the normal kurtosis of 3:
Conclusion
Kurtosis, along with skewness, is one of the variables that measures the normality of a distribution. In a standard normal distribution, kurtosis is 3, which is why a good starting point in statistical interpretation is to determine whether the kurtosis of your distribution differs significantly from 3.
BridgeText can help you with all of your statistical analysis needs.
